Question

For 𝛼 > 0, let $𝑦_{𝛼} (𝑥)$ be the solution to the differential equation
$2 \frac{d^{2} y}{d x^{2}} - \frac{d y}{d x} - y = 0$
satisfying the conditions
$𝑦 (0) = 1, 𝑦' (0) = 𝛼 .$
Then, the smallest value of 𝛼 for which $𝑦_{𝛼} (𝑥)$ has no critical points in ℝ equals
_____________ (rounded off to the nearest integer).

Answer: $\underset{_____________}{1}$

Solution :-

Given that $2 \frac{d^{2} y}{d x^{2}} - \frac{d y}{d x} - y = 0$ this is a 2nd order homogeneous linear differential equation with constant cofficient and we know that general solution of heigher order homogeneous linear differential equation is $y = C . F . (complementary function) .$
Auxiliary Equation of given differential equation is

\begin{array}{crcl} 2 D^{2} - D - 1 & = & 0 \\ ⟹ & 2 D^{2} - 2 D + D - 1 & = & 0 \\ ⟹ & 2 D (D - 1) + 1 (D - 1) & = & 0 \\ ⟹ & (2 D + 1) (D - 1) & = & 0 \\ ∴ & D & = & - \frac{1}{2}, 1. \end{array}

Roots of Auxiliary equation is real and distinct.
Hence, $C . F . = c_{1} e^{- \frac{1}{2} x} + c_{2} e^{x}$

So, the general solution is $y (x) = c_{1} e^{- \frac{1}{2} x} + c_{2} e^{x} .$

Now, given that $y (0) = 1$ , hence from the general solution we get

\begin{array}{llllllllll} 1 & = & c_{1} e^{- \frac{1}{2} \cdot 0} + c_{2} e^{0} \\ ⟹ & 1 & = & c_{1} + c_{2} \\ ∴ & c_{1} + c_{2} & = & 1 \dots \dots (1) \end{array}

\begin{array}{llllllllll} Now, & y^{'} (x) & = & - \frac{1}{2} c_{1} e^{- \frac{1}{2} x} + c_{2} e^{x} \\ ⟹ & α & = & - \frac{1}{2} c_{1} e^{- \frac{1}{2} \cdot 0} + c_{2} e^{0} (given y^{'} (0) = α) \\ ⟹ & α & = & - \frac{1}{2} c_{1} + c_{2} \\ ∴ & - \frac{1}{2} c_{1} + c_{2} & = & α \dots \dots (2) \end{array}

Now, substracting eq(2) from eq(1), we get

\begin{array}{llllllllll} c_{1} + \frac{1}{2} c_{1} & = & 1 - α \\ ⟹ & \frac{3}{2} c_{1} & = & 1 - α \\ ∴ & c_{1} & = & \frac{2}{3} (1 - α) \end{array}

putting the value of $c_{1}$ in eq(1), we get

\begin{array}{llllllllll} \frac{2}{3} (1 - α) + c_{2} & = & 1 \\ ⟹ & c_{2} & = & 1 - \frac{2}{3} (1 - α) \\ ⟹ & c_{2} & = & \frac{3 - 2 (1 - α)}{3} \\ ∴ & c_{2} & = & \frac{1 + 2 α}{3} \end{array}

Now, put the value of $c_{1}$ and $c_{2}$ in the general solution we get final solution as

y_{α} (x) = \frac{2 (1 - α)}{3} \cdot e^{- \frac{1}{2} x} + \frac{(1 + 2 α)}{3} \cdot e^{x} .

Now, for the critical points we have to find first derivative of $y_{α} (x)$ and put equal to zero and then find the value of $α$ for which $x$ is defined.

y_{α}^{'} (x) = \frac{2 (1 - α)}{3} (- \frac{1}{2}) \cdot e^{- \frac{1}{2} x} + \frac{(1 + 2 α)}{3} \cdot e^{x}

For critical points

\begin{array}{crcl} y_{α}^{'} (x) & = & 0 \\ ⟹ & \frac{2 (1 - α)}{3} (- \frac{1}{2}) \cdot e^{- \frac{1}{2} x} + \frac{(1 + 2 α)}{3} \cdot e^{x} & = & 0 \\ ⟹ & - \frac{(1 - α)}{3} \cdot e^{- \frac{1}{2} x} + \frac{(1 + 2 α)}{3} \cdot e^{x} & = & 0 \\ ⟹ & \frac{(1 + 2 α)}{3} \cdot e^{x} & = & \frac{(1 - α)}{3} \cdot e^{- \frac{1}{2} x} \\ ⟹ & e^{\frac{3}{2} x} & = & \frac{(1 - α)}{3} \cdot \frac{3}{(1 + 2 α)} \\ ⟹ & \frac{3}{2} x & = & log (\frac{1 - α}{1 + 2 α}) \\ ∴ & x & = & \frac{2}{3} log (\frac{1 - α}{1 + 2 α}) . \end{array}

Clearly, we can see that $x$ is critical point only if $\frac{1 - α}{1 + 2 α} > 0 ⟹ - \frac{α - 1}{1 + 2 α} > 0$ , here we can apply number line rule for finding domain for $α$ for which $- \frac{α - 1}{1 + 2 α} > 0.$

$α - 1 = 0 ⟹ α = 1$ and $1 + 2 α = 0 ⟹ α = - \frac{1}{2} .$

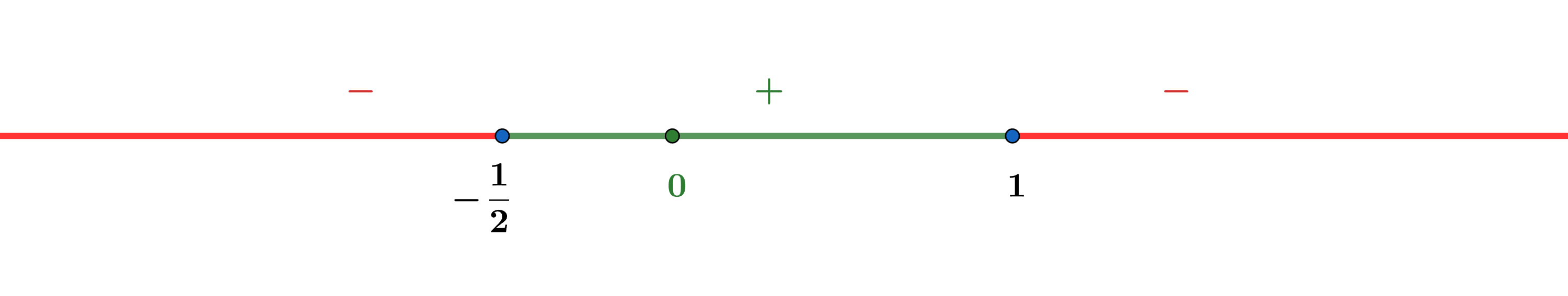
We can see in figure above $α \in (- \frac{1}{2}, 1) .$

Hence, $x$ is a critical point only if $α \in (- \frac{1}{2}, 1)$ and also given that $α > 0$ hence the interval for $α$ is reduced to $(0, 1)$ and for all $α \in (0, 1)$ we get $x$ as critical point but if $α \in [1, \infty)$ , we do not get any critical point.

Therefore, the smallest value of 𝛼 for which $𝑦_{𝛼} (𝑥)$ has no critical points in ℝ equals $1.$

So, the correct answer is $\to$ $\underset{_____________}{1}$

#Ordinary Differential Equation #IIT JAM PYQ 2024 #Mathematics #IIT JAM PYQs